Bernoulli Roulette System
Because flowing blood has mass and velocity it has kinetic energy (KE). This KE is proportionate to the mean velocity squared (V2; from KE = ½ mV2). Furthermore, as the blood flows inside a vessel, pressure is exerted laterally against the walls of the vessel; this pressure represents the potential or pressure energy (PE). The total energy (E) of the blood flowing within the vessel, therefore, is the sum of the kinetic and potential energies (assuming no gravitational effects) as shown below.
E = KE + PE (where KE ∝ V2)Therefore, E ∝ V2 + PE
There are two important concepts that follow from this relationship.
- Blood flow is driven by the difference in total energy between two points. Although pressure is normally considered as the driving force for blood flow, in reality it is the total energy that drives flow between two points (e.g., longitudinally along a blood vessel or across a heart valve). Throughout most of the cardiovascular system, KE is relatively low, so for practical purposes, it is stated that the pressure energy (PE) difference drives flow. When KE is high, however, adding KE to the PE significantly increases the total energy, E. To illustrate this, consider the flow across the aortic valve during cardiac ejection. Late during ejection, the intraventricular pressure (PE) falls slightly below the aortic pressure (PE), nevertheless, flow continues to be ejected into the aorta. The reason for this is that the KE of the blood as it moves across the valve at a very high velocity ensures that the total energy (E) in the blood crossing the valve is higher than the total energy of the blood more distal in the aorta.
- Kinetic energy and pressure energy can be interconverted so that total energy remains unchanged. This is the basis of Bernoulli's Principle. This principle can be illustrated by a blood vessel that is suddenly narrowed then returned to its normal diameter. In the narrowed region (stenosis), the velocity increases as the diameter decreases. Quantitatively, V ∝ 1/D2 because flow (F) is the product of mean velocity (V) and vessel cross-sectional area (A) (F = V x A), and A is directly related to diameter (D) (or radius, r) squared (from A = π r2). If the diameter is reduced by one-half in the region of the stenosis, the velocity increases 4-fold. Because KE ∝ V2, the KE increases 16-fold. Assuming that the total energy is conserved within the stenosis (E actually decreases because of resistance as shown in the figure), then the 16-fold increase in KE must result in a reciprocal decrease in in the magnitude of PE. Once past the narrowed segment, KE will revert back to its pre-stenosis value because the post-stenosis diameter is the same as the pre-stenosis diameter and flow (and therefore, velocity) is conserved. Because of the resistance of the stenosis, and the turbulence the likely occurs, the post-stenosis PE and E will both fall. To summarize this concept, blood flowing at higher velocities has a higher ratio of kinetic energy to potential (pressure) energy.
Bernoulli’s equation is, in fact, just a convenient statement of conservation of energy for an incompressible fluid in the absence of friction. Making Connections: Conservation of Energy Conservation of energy applied to fluid flow produces Bernoulli’s equation.
An interesting, yet practical application of Bernoulli's Principle is found when blood pressure measurements are made from within the ascending aorta. As described above, during ventricular ejection, the velocity and hence kinetic energy of the flowing blood is very high. The instantaneous blood pressure that is measured within the aorta will be significantly different depending upon how the pressure is measured. As illustrated to the right, if a catheter has an end-port (E) sensor that is facing the flowing stream of blood, it will measure a pressure that is significantly higher than the pressure measured by a side-port (S) sensor on the same catheter. The reason for the discrepancy in measured pressures is that the end-port measures the total energy of the flowing blood. As the flow stream 'hits' the end of the catheter, the kinetic energy (which is high) is converted to potential (or pressure) energy, and added to the potential energy to equal the total energy. The side-port will not be 'hit' by the flowing stream so kinetic energy is not converted to potential energy. The side-port sensor, therefore, only measures the potential energy, which is the lateral pressure acting on the walls of the aorta. The difference between the two types of pressure measurements can range from a few mmHg to more than 20 mmHg depending upon the peak velocity of the flowing blood within the aorta.
Revised 01/27/20
The law of large Numbers was established in the 17th century by Jacob Bernoulli showing that the larger the sample of an event - like a coin toss - the more likely it is to represent its true probability. Bettors still struggle with this idea 400 years on which is why it has become known as the gambler’s fallacy. Find out why this mistake can be so costly.
- Bernoulli and Binomial Page 8 of 19. The Bernoulli Distribution. Note – The next 3 pages are nearly. Identical to pages 31-32 of Unit 2, Introduction to Probability. They are reproduced here for ease of reading. The Bernoulli Distribution is an example of a discrete probability distribution.
- The Bernoulli trials process, named after Jacob Bernoulli, is one of the simplest yet most important random processes in probability. Essentially, the process is the mathematical abstraction of coin tossing, but because of its wide applicability, it is usually stated in terms of a sequence of generic trials.
The law of large numbers

Using a fair coin toss as an example (where the chance of hitting heads and tails has an equal 50% chance), Bernoulli calculated that as the number of coin tosses gets larger, the percentage of heads or tails results gets closer to 50%, while the difference between the actual number of heads or tails thrown also gets larger.
Bernoulli Roulette System Using
'As the number of tosses get larger the distribution of heads or tails evens out to 50%'
It’s the second part of Bernoulli’s theorem that people have a problem understanding – which has led to it being coined the “Gambler’s Fallacy”. If you tell someone that a coin has been flipped nine times, landing on heads each time, their prediction for the next flip tends to be tails.
This is incorrect, however, as a coin has no memory, so each time it is tossed the probability of heads or tails is the same: 0.5 (a 50% chance).
Bernoulli’s discovery showed that as a sample of fair coin-tosses gets really big – e.g. a million – the distribution of heads or tails would even out to around 50%. Because the sample is so large, however, the expected deviation from an equal 50/50 split can be as large as 500.
This equation for calculating the statistical standard deviation gives us an idea what we should expect:
While the expected deviation is observable for this many tosses, the nine-toss example mentioned earlier isn’t a large enough sample for this to apply.
Therefore the nine tosses are like an extract from the million-toss sequence – the sample is too small to even-out like Bernoulli suggests will happen over a sample of a million tosses, and instead can form a sequence by pure chance.
Applying distribution in betting
There are some clear applications for expected deviation in relation to betting. The most obvious application is for casino games like Roulette, where a misplaced belief that sequences of red or black or odd or even will even out during a single session of play can leave you out of pocket. That’s why the Gambler’s Fallacy is also known as the Monte Carlo fallacy.

In 1913, a roulette table in a Monte Carlo casino saw black come up 26 times in a row. After the 15th black, bettors were piling onto red, assuming the chances of yet another black number were becoming astronomical, thereby illustrating an irrational belief that one spin somehow influences the next.
'In 1913, a roulette table in a Monte Carlo casino saw black come up 26 times in a row. For that reason, gambler’s fallacy is also known as Monte Carlo fallacy'
Bernoulli Roulette System Review
Another example could be a slot machine, which is in effect a random number generator with a set RTP (Return to Player). You can often witness players who have pumped considerable sums into a machine without success embargoing other players from their machine, convinced that a big win must logically follow their losing run.
Of course, for this tactic to be viable, the bettor would have to have played an impractically large number of times to reach the RTP.
When he established his law, Jacob Bernouilli asserted that even the stupidest man understands that the larger the sample, the more likely it is to represent the true probability of the observed event. He may have been a little harsh in his assessment by once you have an understanding of the Law of Large Numbers, and the law (or flaw) of averages is consigned to the rubbish bin, you won’t be one of Bernouilli’s ‘stupid men’.
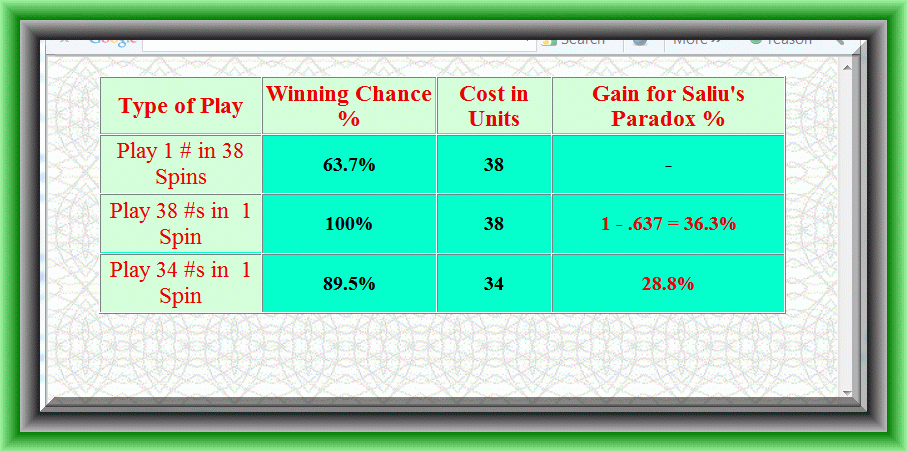
Bernoulli Roulette System Chart
If you enjoyed this content you may be interested in Pinnacle's betting psychology articles.